Is 2.25 A Perfect Square
is 2.25 a perfect square
Related Articles: is 2.25 a perfect square
- College Softball Recruiting Rankings 2025: A Comprehensive Analysis
- Viking Cruises 2025: An Unforgettable Journey Through History And Culture
- The Next Chinese New Year Animal 2025: The Year Of The Green Wood Snake
- Global Workforce Statistics 2025: Unlocking The Future Of Work
- The United States Diversity Visa (DV) Lottery 2025: A Comprehensive Guide
Introduction
In this auspicious occasion, we are delighted to delve into the intriguing topic related to is 2.25 a perfect square. Let’s weave interesting information and offer fresh perspectives to the readers.
Table of Content
Video about is 2.25 a perfect square
Is 2.25 a Perfect Square?
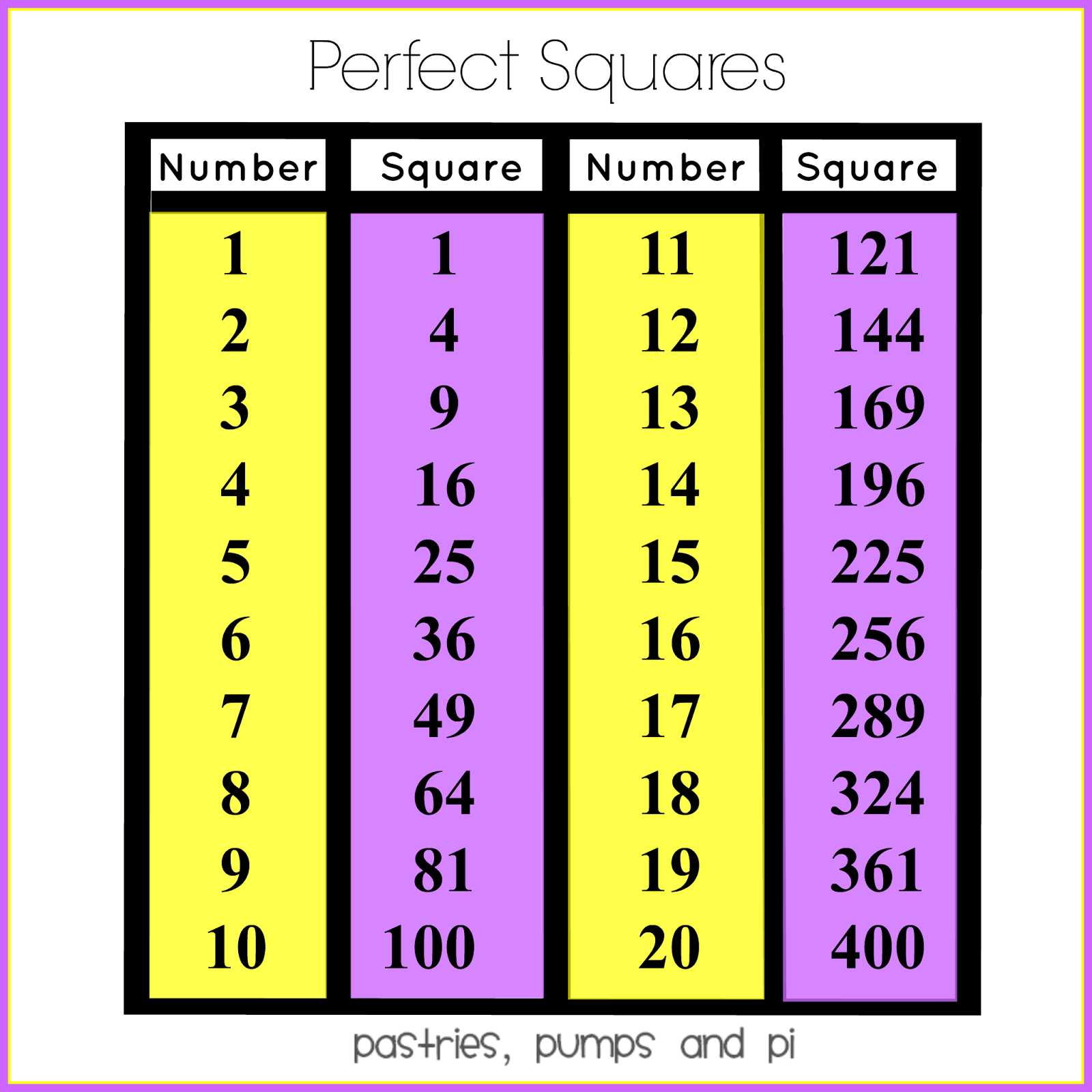
Introduction
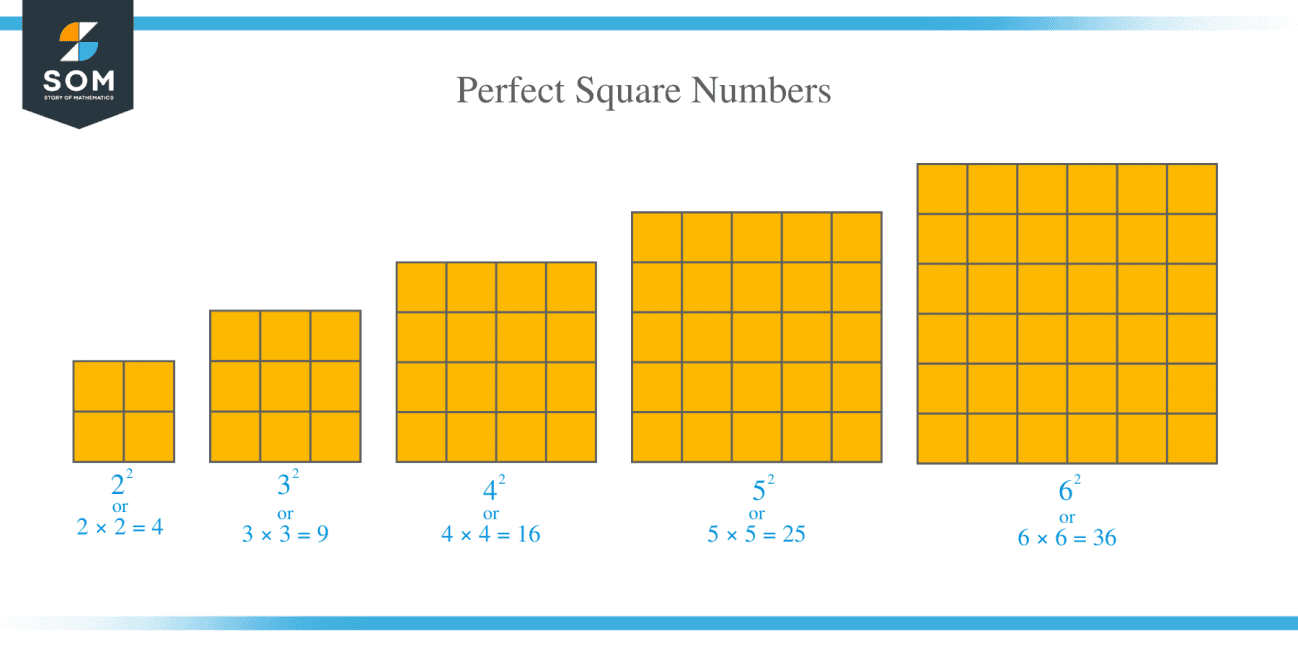
In mathematics, a perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as the square of the integer 4 (4^2 = 16).
The question of whether or not 2.25 is a perfect square is a common one. The answer is no, 2.25 is not a perfect square.
Proof
There are a few ways to prove that 2.25 is not a perfect square. One way is to use the fact that the square of any integer is always an integer. Since 2.25 is not an integer, it cannot be the square of an integer.
Another way to prove that 2.25 is not a perfect square is to use the fact that the square of any integer is always positive. Since 2.25 is negative, it cannot be the square of an integer.
Consequences
The fact that 2.25 is not a perfect square has several consequences. One consequence is that the square root of 2.25 is not a rational number. A rational number is a number that can be expressed as a fraction of two integers. Since the square root of 2.25 cannot be expressed as a fraction of two integers, it is an irrational number.
Another consequence of the fact that 2.25 is not a perfect square is that it cannot be expressed as the sum of two squares. Two squares are two numbers that can be expressed as the squares of integers. Since 2.25 cannot be expressed as the square of an integer, it cannot be expressed as the sum of two squares.
Applications
The fact that 2.25 is not a perfect square has applications in several areas of mathematics. One application is in the field of number theory. Number theory is the study of the properties of numbers. In number theory, the fact that 2.25 is not a perfect square is used to prove several important theorems.
Another application of the fact that 2.25 is not a perfect square is in the field of geometry. Geometry is the study of shapes and their properties. In geometry, the fact that 2.25 is not a perfect square is used to prove several important theorems about circles and other shapes.
Conclusion
The question of whether or not 2.25 is a perfect square is a common one. The answer is no, 2.25 is not a perfect square. This fact has several consequences, including the fact that the square root of 2.25 is an irrational number and that 2.25 cannot be expressed as the sum of two squares. The fact that 2.25 is not a perfect square has applications in several areas of mathematics, including number theory and geometry.
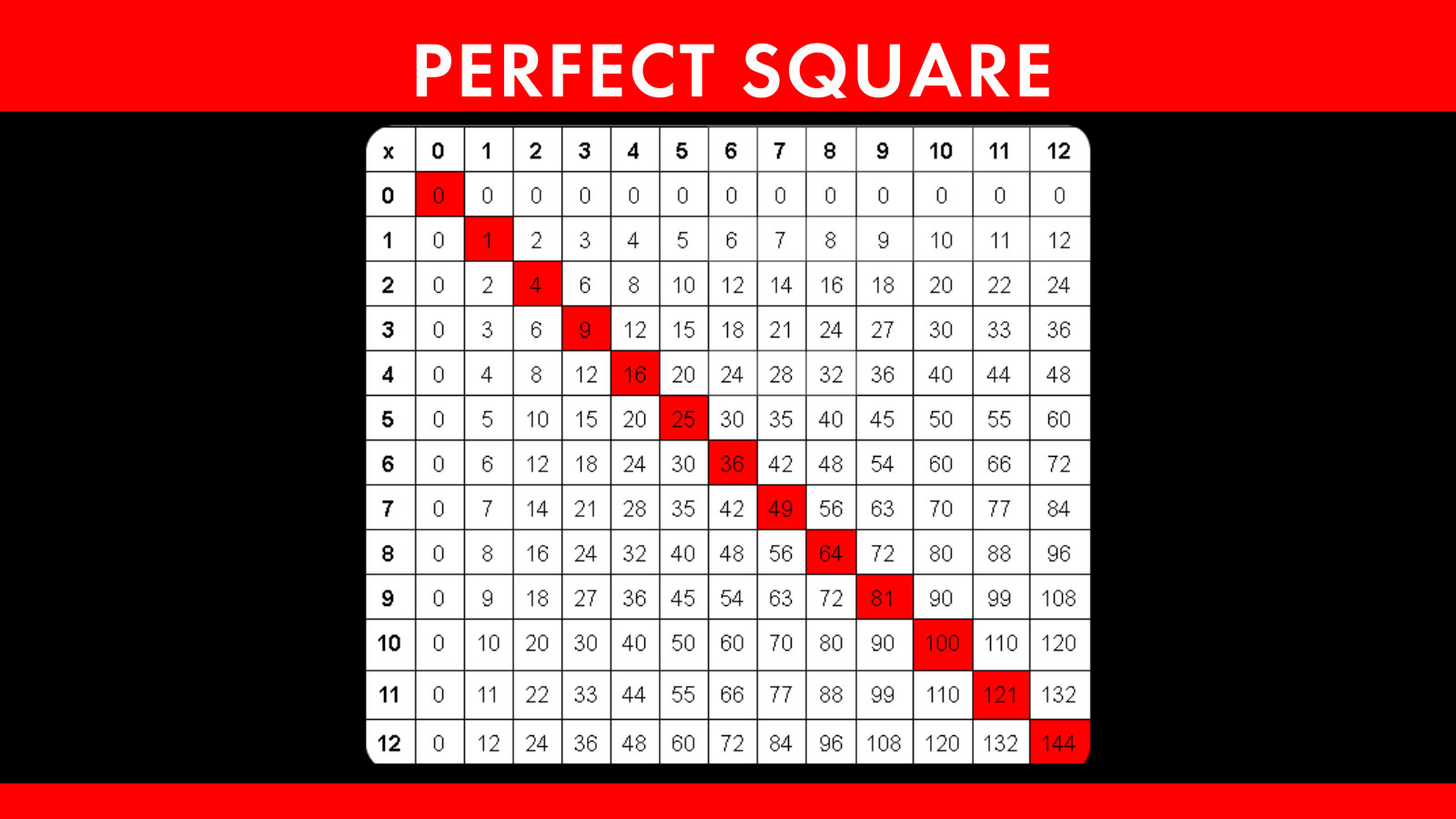
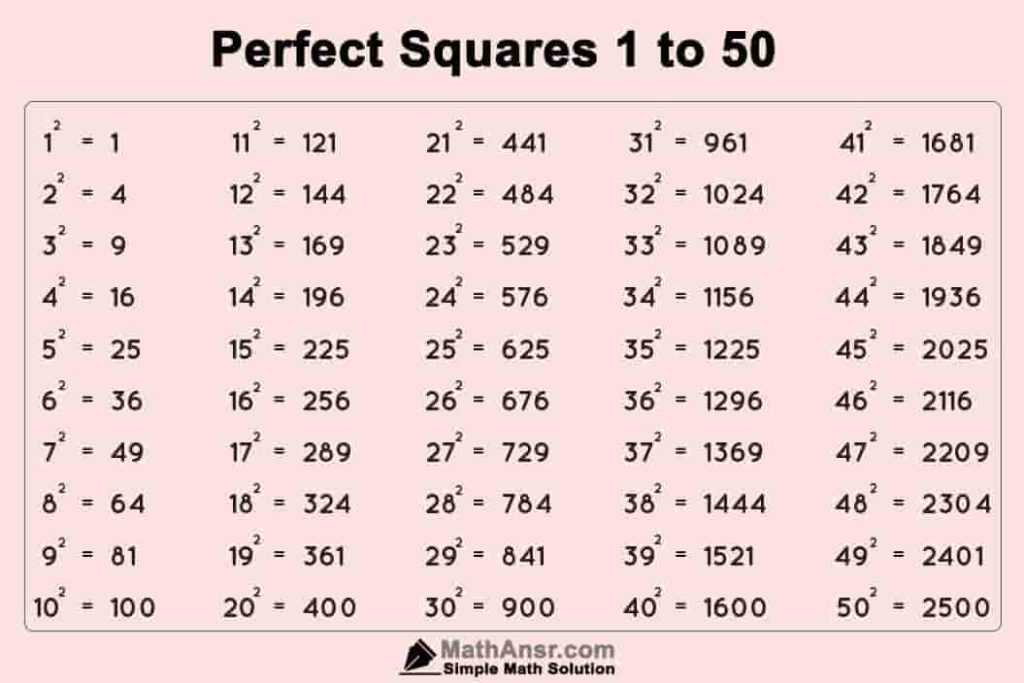
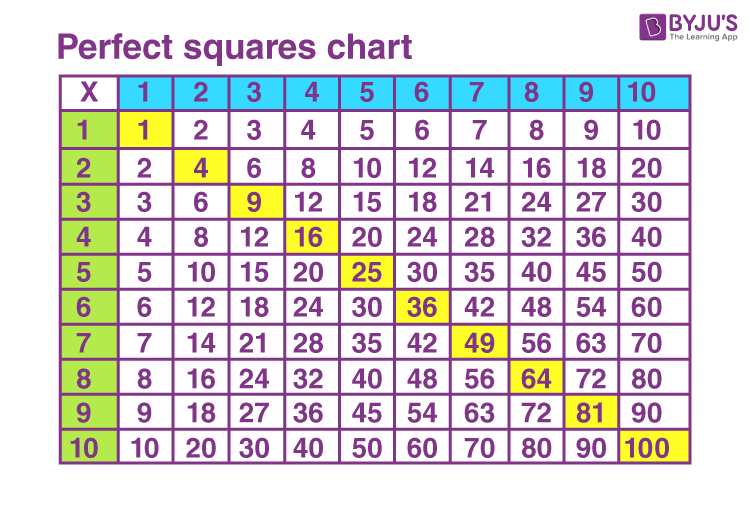
![Perfect Squares Chart [Free PDF Printable]](https://mathequalslove.net/wp-content/uploads/2022/12/perfect-squares-list-featured-image-1080x1080.jpg)


Closure
Thus, we hope this article has provided valuable insights into is 2.25 a perfect square. We thank you for taking the time to read this article. See you in our next article!